1.Database management system - is: 2. In Access, the basic object is:
3. In Access, the line is called:
4. In Access, a column called:
5. A structure of a table is:
6. In Access, there is a data type:
7. The process of creating a table in Access by choosing from existing fields: (The template tables)
8. In Access, in the template table fields delete button of the selected fields: (<.)
9. In Access, you can enter to create a table in Design view:
10. In Access field names are allowed to:
11. In Access to the field names must not contain:
12. In Access, the key field is used to:
13. In Access mode allows you to change the layout of the table, query, report:
14. Sorting is:
15. The logical OR operand is:
16. The logical operand AND means:
17. The logical operand NOT means:
18. In what menu item in Access there is a Filter: (Entries)
19. In Access, the number of types of links:
20. To link the tables use the command:
21. In Access possible to establish a connection between the tables:
22. In Access the proportion (1 - 1) corresponds to the relationship:
23. In Access the proportion (∞ - 1) corresponds to the relationship:
24. In Access sampling means is:
25. In Access to include the desired field in the request form should be:
26. In Access Expression Builder is used to:
27. In Access field names in calculated fields should be taken:
28. In Access function COUNT:
29. The Access operation of calculating the mean value:
30. In Access, the main difference between AutoForm regime in the column of the table mode:
Обозначим C(n) - число набрать n рублей. Очевидно, C(n) = C(n-1) + C(n-2) + C(n-5) + C(n-10) [Представим себе. что мы знаем число набрать n-5 рублей. Тогда если мы уверены, что последней вытащили 5-рублёвую монету, то будет C(n). Финальный ответ - сумма по всем возможным выборам последней монеты]
Полагая C(n) = 0 при всех n < 0, C(0) = 1, получим по этой формуле
С(66) = 1431020833989040
Cчитать можно, например, такой программой:
var C: array[-9..66] of BigInteger;
begin
for var i := -9 to -1 do
C[i] := 0;
C[0] := 1;
for var i := 1 to 66 do
C[i] := C[i - 1] + C[i - 2] + C[i - 5] + C[i - 10];
print(C[66]);
end.
Вариант понимания условия №2. Нам порядок выдачи не важен. Тогда вопрос по сути сводится к числу целых неотрицательных решений уравнения
x + 2y + 5z + 10t = 66, где x, y, z, t - число 1-, 2-, 5- и 10-рублёвых монет соответственно.
Тут можно написать общую формулу, но она будет объемной, так что вычислять по ней совсем не радостно (даже с компьютером). Поэтому проще все варианты перебрать. ответ получится 700.
Пример программы:
begin
var count := 0;
for var t := 0 to 6 do
for var z := 0 to (66 - 10*t) div 5 do
for var y := 0 to (66 - 10*t - 5*z) div 2 do
inc(count);
print(count);
end.
Подобным образом можно считать и вручную. По сути нам требуется вычислить сумму [1 + (66 - 10t - 5z)/2] по всем допустимым t, z ([x] - целая часть x). Перебираем сначала t, потом z:
t = 0. z = 0,1,2,...,13. Вклад в сумму 34 + 31 + 29 + 26 + 24 + 21 + 19 + 16 + 14 + 11 + 9 + 6 + 4 + 1 = 245.
t = 1. z = 0,1,2,...,11. Легко понять, что здесь будут все числа без первых двух слагаемых: 29 + 26 + 24 + 21 + 19 + 16 + 14 + 11 + 9 + 6 + 4 + 1 = 245 - 34 - 31 = 180
Аналогично, t = 2: 180 - 29 - 26 = 125
t = 3: 125 - 26 - 21 = 80
t = 4: 80 - 19 - 16 = 45
t = 5: 45 - 14 - 11 = 20
t = 6: 20 - 9 - 6 = 5
Итого 245 + 180 + 125 + 80 + 45 + 20 + 5 = 700
Каждый следующий член с знаменателем n! получается умножением предыдущего на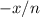
. Очевидно, последовательность убывает по модулю, так что достаточно пройтись циклом, и, если новый вычисленный член по модулю меньше 0,0001, остановиться.
Окажется, что уже пятый член меньше ε, так что выведется только 4 числа.
Код:
#include <iostream>
#include <cmath>
int main() {
const double x = 0.5;
const double eps = 0.0001;
double term = x * x / 2;
for (int n = 3; std::abs(term) > eps; n++) {
std::cout << term << " ";
term *= -x/n;
}
}
Вывод:
0.125 -0.0208333 0.00260417 -0.000260417